We have been working on a prototype of three-dimensional FDEM. We’ve implemented most of the critical features into the code, including a quasi-static friction law, Mohr-Coulomb failure criterion, and transversely isotropic elastic constitutive law.

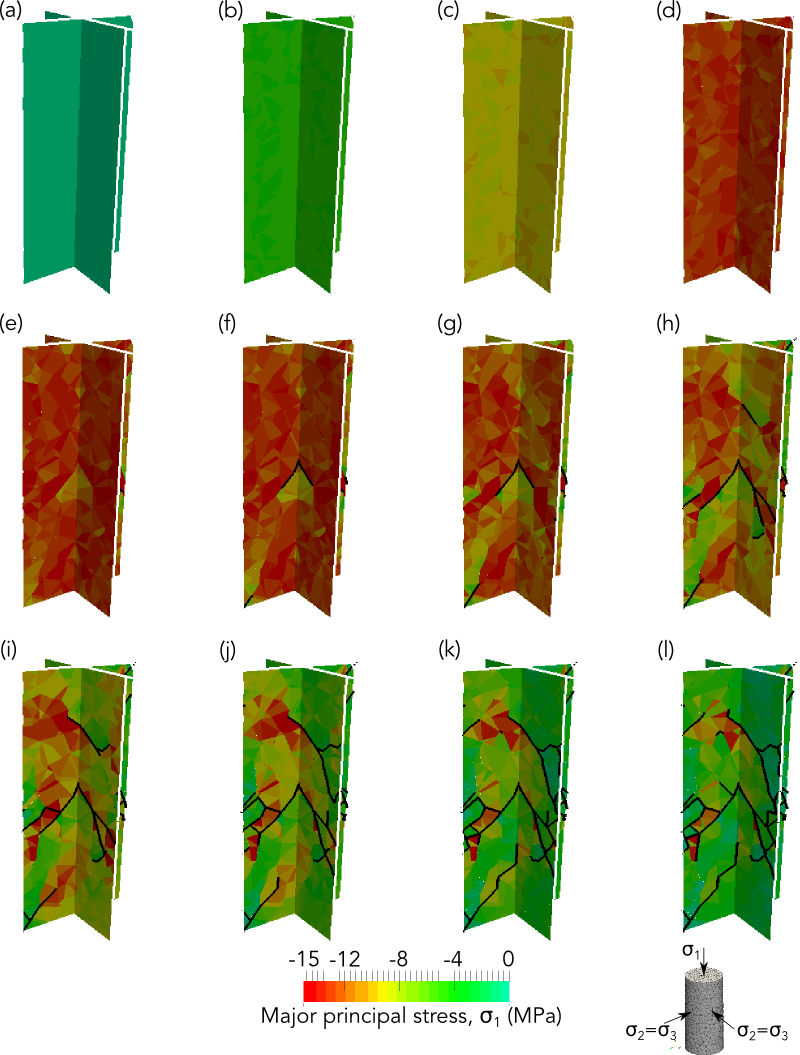
We’re excited to showcase some of the simulations results here. The figure below shows the simulation of a uniaxial compression test representing a mine pillar. The model is capable of capturing the progressive damage of the rock from isolated fractures that coalesce to form macroscopic fractures. The overall strength of the rock closely matches laboratory results.

Major principal stress and fracture pattern of a 3D uniaxial compression test resembling a mine pilar.

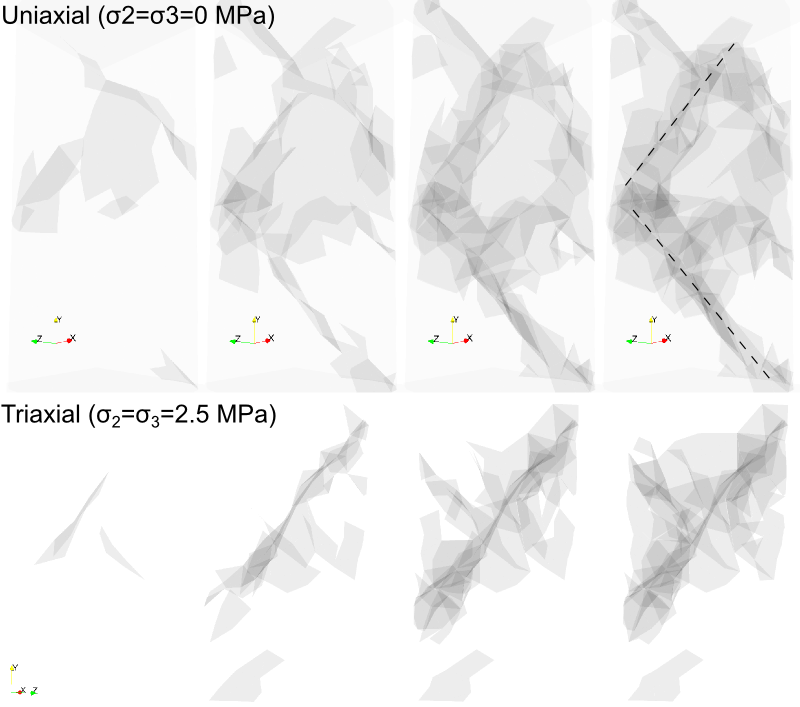
X-ray style 3D fracture volume evolution of the rock is also shown below for an unconfined uniaxial simulation (σ₃=0) and a confined triaxial test (σ₂=σ₃=2.5 MPa). The grey-scale intensity in the images represents fracture density (i.e., higher density corresponds to more localized fractures). As shown in the top section of the figure, the fractures form a major plane of failure at about 52°, which corresponds well with the theoretical Mohr-Coulomb value (45° + 0.5 * friction angle). As the bottom section shows, the addition of a small confining pressure (σ₃=2.5 MPa) localizes the fractures further into a shear band.

3D fracture volume evolution of a uniaxial compression test and a triaxial test with σ₃=2.5 MPa.

More 3D results will be showcased in following blog posts.